

In addition, the change in x 3 x 3 forcing a change in sin ( x 3 ) sin ( x 3 ) suggests that the derivative of sin ( u ) sin ( u ) with respect to u, u, where u = x 3, u = x 3, is also part of the final derivative. First of all, a change in x x forcing a change in x 3 x 3 suggests that somehow the derivative of x 3 x 3 is involved. This chain reaction gives us hints as to what is involved in computing the derivative of sin ( x 3 ). We can think of this event as a chain reaction: As x x changes, x 3 x 3 changes, which leads to a change in sin ( x 3 ). Consequently, we want to know how sin ( x 3 ) sin ( x 3 ) changes as x x changes. We can think of the derivative of this function with respect to x as the rate of change of sin ( x 3 ) sin ( x 3 ) relative to the change in x. To put this rule into context, let’s take a look at an example: h ( x ) = sin ( x 3 ). Instead, we use the chain rule, which states that the derivative of a composite function is the derivative of the outer function evaluated at the inner function times the derivative of the inner function. However, using all of those techniques to break down a function into simpler parts that we are able to differentiate can get cumbersome. When we have a function that is a composition of two or more functions, we could use all of the techniques we have already learned to differentiate it. In this section, we study the rule for finding the derivative of the composition of two or more functions.

However, these techniques do not allow us to differentiate compositions of functions, such as h ( x ) = sin ( x 3 ) h ( x ) = sin ( x 3 ) or k ( x ) = 3 x 2 + 1. ) as well as sums, differences, products, quotients, and constant multiples of these functions. We have seen the techniques for differentiating basic functions ( x n, sin x, cos x, etc. 3.6.5 Describe the proof of the chain rule.3.6.4 Recognize the chain rule for a composition of three or more functions.3.6.3 Apply the chain rule and the product/quotient rules correctly in combination when both are necessary.3.6.2 Apply the chain rule together with the power rule.
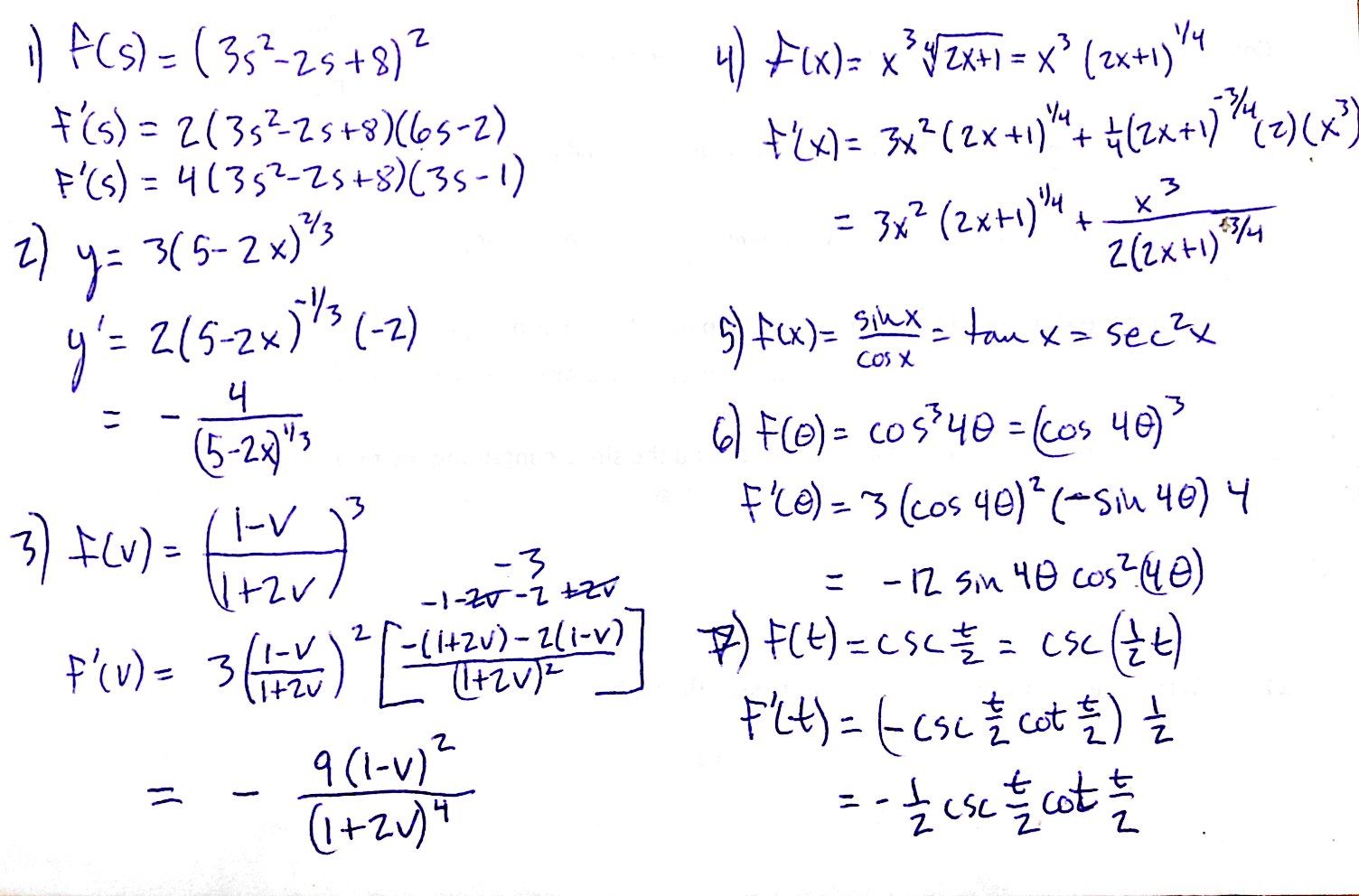


 0 kommentar(er)
0 kommentar(er)
